contact : Marion Delehaye (marion.delehaye@femto-st.fr)
Ultra-stable superradiant laser based on ytterbium atoms
This project aims at the realization of an ultra-stable superradiant laser. Superradiant lasers unify two major systems widely used in time and frequency metrology: an ultra-stable optical Fabry-Perot cavity, and an ultracold atomic sample.
Context
Time and frequency metrology historically relied on the observation of the position of Earth with respect to other celestial bodies. It underwent several disruptions during the past decades, starting with the redefinition of the SI second in 1967 based on electronics resonances in cesium atom. Since then, advances made in time and frequency metrology with atomic clocks have made them versatile and multidisciplinary tools not only for the measurement of time, but also for a broad variety of applications, ranging from telecommunications to fundamental physics tests.
The current best timekeepers are constituted of a local oscillator, here a laser pre-stabilized on an optical Fabry-Perot cavity, that is locked on an atomic sample which is either an ultracold neutral atom ensemble or a trapped ion. This type of clocks is thus called passive optical clock.
The operation of active optical clocks has been demonstrated recently[1]. They rely on the superradiant radiation emitted by an atomic ensemble in an optical cavity, without external laser. Pioneering work reported in [3] has led to the observation of ultra-stable light pulses emitted by a superradiant strontium cloud . It is expected that superradiant lasers could have a fractional frequency stability as low as 10-18 τ-1/2 [2].
Description of the project
We are realizing a superradiant laser based on ytterbium atoms, in order to obtain a continuous ultra-stable signal. Ultracold (10 μK) 171Yb atoms will be held in an optical lattice and located inside an ultra-stable optical Fabry-Perot cavity (σy(τ) = 10-14).
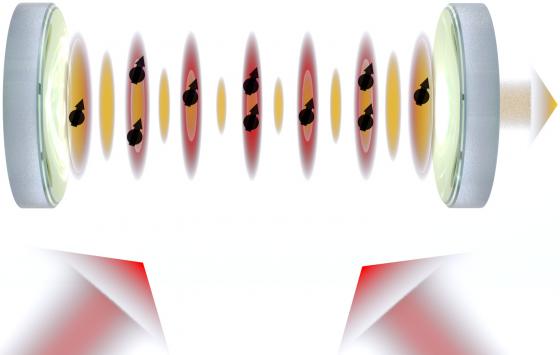
Superradiance is a collective atomic emission phenomenon that occurs when N atomic dipoles are forced to oscillate in phase by an electromagnetic wave. The emitted intensity is then proportional to N2 rather than to N, as it would be the case for independent dipoles. Historically, this phenomenon has first been described in the case when the atoms are prepared in an excited state and held in a volume with dimensions smaller than the emission wavelength λ0 . This condition ensures coupling between the atomic dipoles through the electromagnetic field and leads to a synchronization of the dipoles. Another way to ensure an in-phase oscillation of the atomic dipoles is to couple them selectively to a single electromagnetic mode, in a cavity of linewidth κ for instance. The coupling g between atoms and the cavity mode then has to be much greater than γ, so that photons are mostly emitted in the cavity mode. In this case, the spectral width of the transition is C γ = g2 /κ and the whole system acts as a “bad-cavity” laser (κ ≫ g ≫ γ). In particular, the emitted photons are more likely to leave the cavity than to be re-absorbed. The resonance of the cavity influences only weakly the frequency of the emitted electromagnetic wave and relaxes the constraints on the cavity stability. This is illustrated in Fig. 1. Using optical pumping, it is possible to maintain a continuous emission, where the linewidth is not limited any more by the duration of the superradiant pulses.
Fig. 1 : Illustration of the working principles of a superradiant laser. N atoms are placed inside an optical Fabry-Perot cavity tuned to the clock transition wavelength in order to maintain in-phase oscillation between the atomic dipoles. Emitted superradiant photons escape the cavity before their re-absorption by other atoms.
References
[1] Superradiance on the millihertz linewidth strontium clock transition, M. A. Norcia, M. N. Winchester, J. R. K. Cline and J. K. Thompson, Science Advances 2, 1601231 (2016).
[2] Prospects for a millihertz-linewidth laser, D. Meiser, Jun Ye, D. R. Carlson, and M. J. Holland, Phys. Rev. Lett. 102, 163601 (2009).
[3] Frequency measurements of superradiance from the strontium clock transition, M. A. Norcia, J. R. K. Cline, J. A. Muniz, J. M. Robinson, R. B. Hutson, A. Goban, G. E. Marti, J. Ye, and J. K. Thompson, Phys. Rev. X 8, 021036 (2018).